knot theories II
-
tri unknot
A line broken by an inner negative space rotates to form an unknot, a continuous line with no overlapping. The component used as the basis for this piece, as well as the resulting structure, can be unraveled to form a loop. Tri Unknot was on display at the McGhee Tyson Airport (TYS) in 2023.
-
tri unicursal knot
A unicursal line is inlaid in copper on the face of a hollow truncated tetrahedron. The knot creates a cognitive visual illusion of three shapes versus two. The illusion that there are sort of 3 shapes but only really 1 is something I like about this one.
-
layered multi polygon knot
A group of three similar polygons rotate to form a knot. Multiple glass layers show colors, the knot itself, and a faint outline.
-
metatronic solid (hexahedron)
Four lines make a reversed knot pattern covering all faces of a hexahedron, regardless of rotation (this same pattern can tile a plane).
-
unicursal hex knot
A self-intersecting line rotates to create a hexagonal shape. The line can be followed from any point all the way around to loop back to its beginning. This was another experiment involving cut paper on charred wood. My first attempt at realizing this design was with wire in encaustic medium, shown here. Even though it…
-
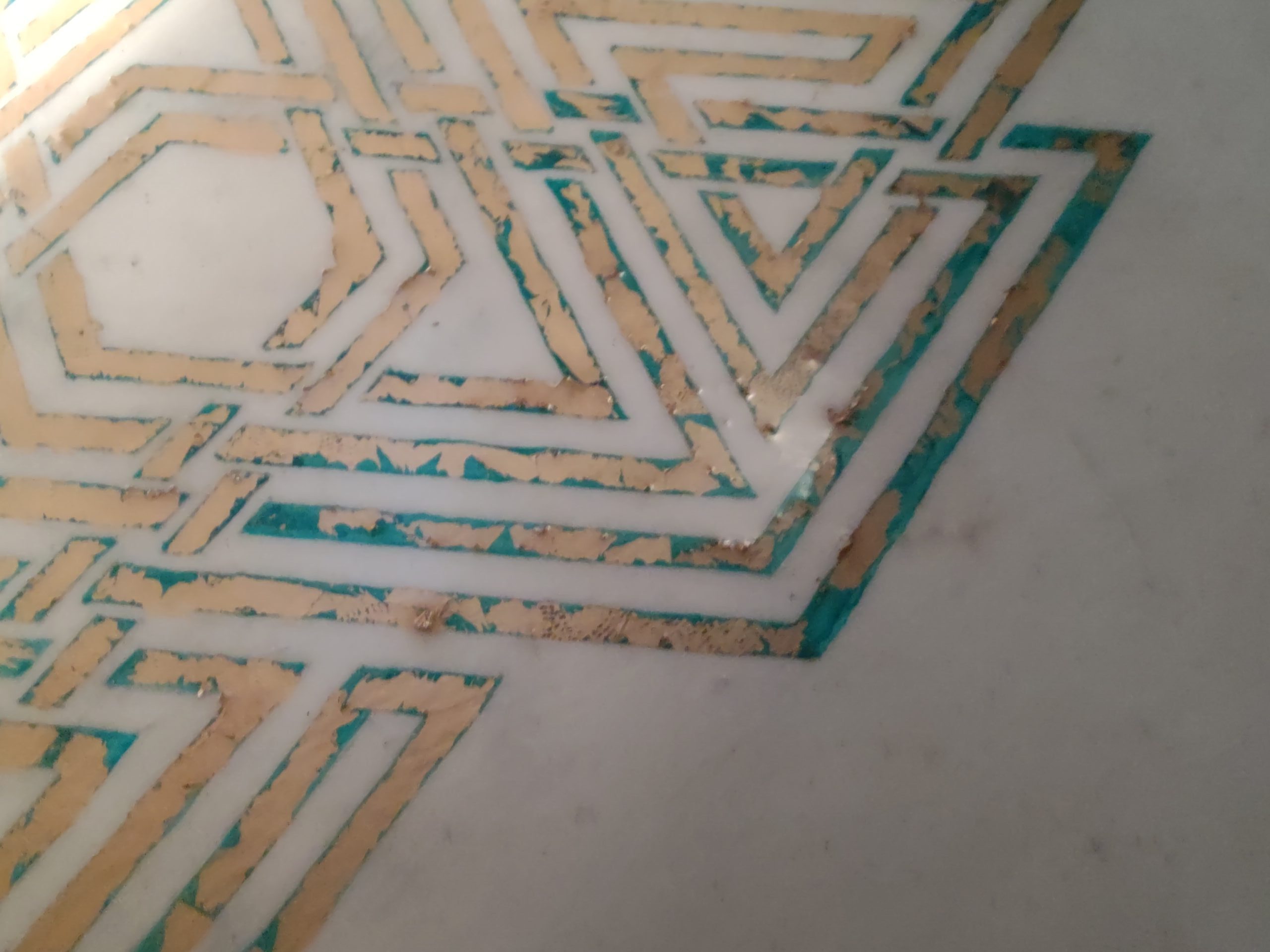
tri angle polygonal knot
A parallelogram and a triangular line rotate to create an overall triangular shape. Encaustic medium covers gilded ink. My first step was putting the main design down in ink. (I phrased the media used in this piece as “Patty Ink” because it once belonged to Pat Lauderdale, someone who always encouraged my artistic endeavors. She…
-
lattice tile
One component of an interlocking tile extends its connectors and uses color variations to imply depth and an isometric perspective. Stained veneer is inlaid in a wood background. This piece involves a bit of experimentation with an isometric knot. The interlocking square shapes require more than one color to give the effect that they are…
-
knot tiling triangle-faced polyhedra
A single shape tiles the faces of all three equilateral triangle-faced regular polyhedra with knots.
-
tile hexahedron
A shape with interlocking edges tiles a plane. The edges of these planes bend to form a hexahedron.
-
scale tile listing band
Twenty-three identical scales are tied together in a continuous loop representing a non-orientable surface. A pattern of accented hexagons covers interlocking segments which twist and bend in an infinite knot.