individual pieces
-
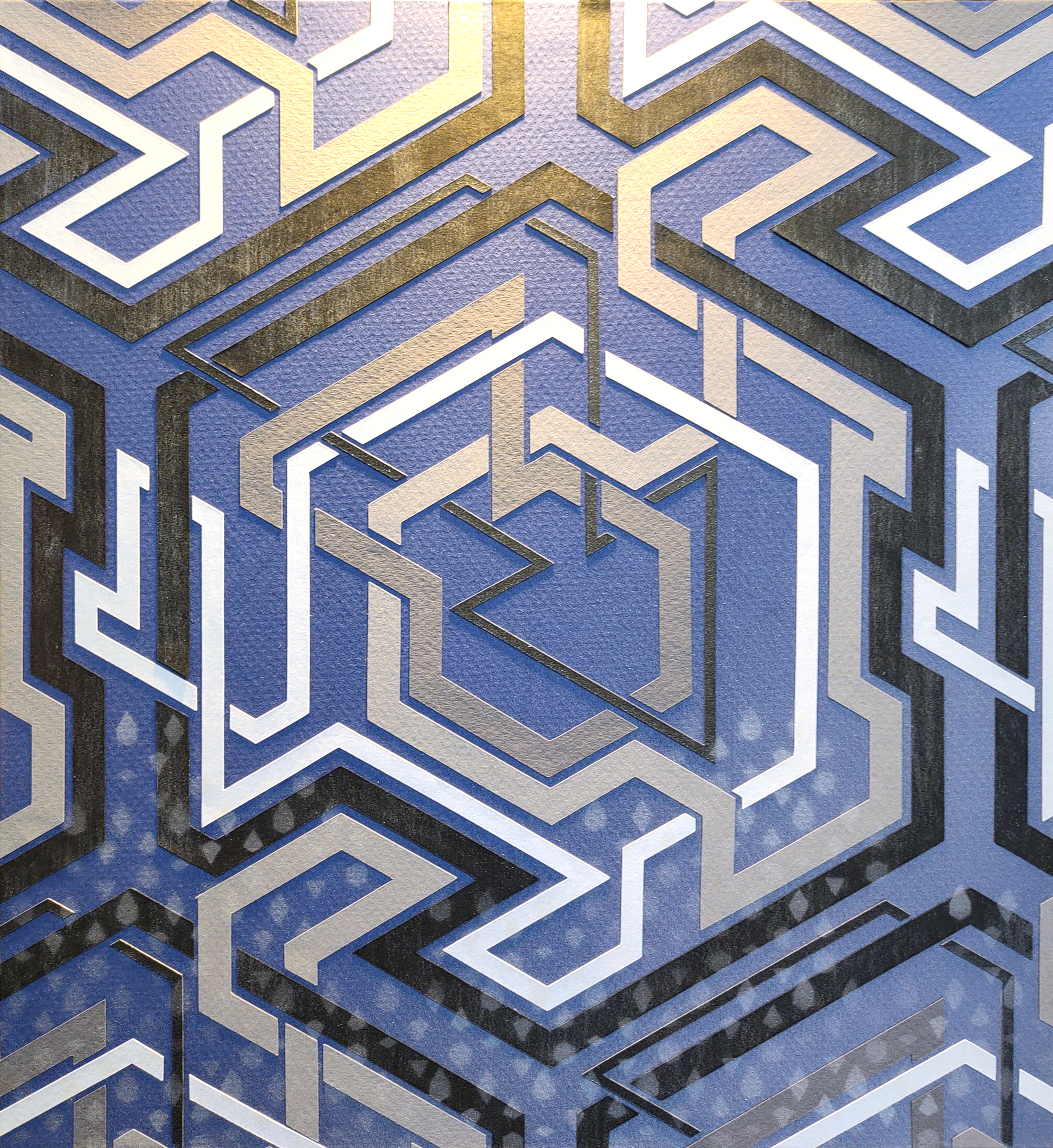
surface-tiling curves
semi-technical description and examples of a new way to use space-filling curves
-
Pool of the Black Star video
short film to accompany music by KillDry
-
hadassah 5783
lattice knot detail in cut paper
-
exploded hexahedron tiled with a plane-filling curve
A single line bisects a hollow cube into two mirrored sections, revealing a second, smaller solid cube inside which supports the three pieces when suspended from the top corner. The bisecting line (curve) is plane-filling, and each face of the exploded hexahedron is identical. A single line bisects a hollow cube into two mirrored sections,…
-
Pool of the Black Star album art
album art and some extras for guitar duo KillDry
-
interlocking panel box
an attempt at making a box with identical interlocking panels
-
hail album art
a variety of album art treatments for my own music
-
sphere of fifths
layers of glass projecting shadows
-
illumination 2014
circular piece with a lot of writing
-
proof
art as a container for art
-
recursive antecedent quick response code experiment
an experiment with QR-ecursion
-
martian / alien painting
a painting full of nostalgia
-
the carnival of the animals puppets
paper puppets with recycled materials
-
my name
Below are real life implementations of a logotype of my first name. The first version of this was created when I was in middle school, c.1990. After taking art more seriously, or at least doing more art, I have been in search of a symbol or mark which represents me. I returned to a version…
-
student work
artwork completed long ago
