Category: partial dimensions
axonometric tiles
Lattices of three different colors intersect over a field of triangles. The large hexagon contains the basic unit for the pattern, made up of transformed copies.
double quad knot
Two knots based on 90° radial symmetry intertwine. The same material (steel) is heat treated with different processes to create two different colors.
negative single twist components
A helix and a hexahedron are both composed from a single component, a negative single twist knot.
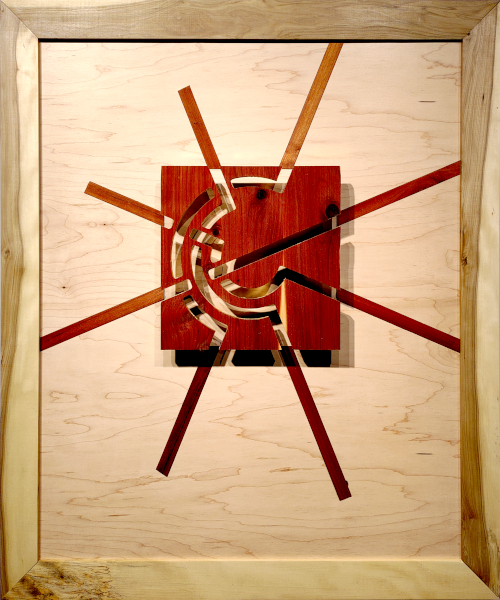
branching unknot
Two unknots are arranged vertically above a charred wood base. The shape of the metal makes self-supporting convex and concave arrangements possible.
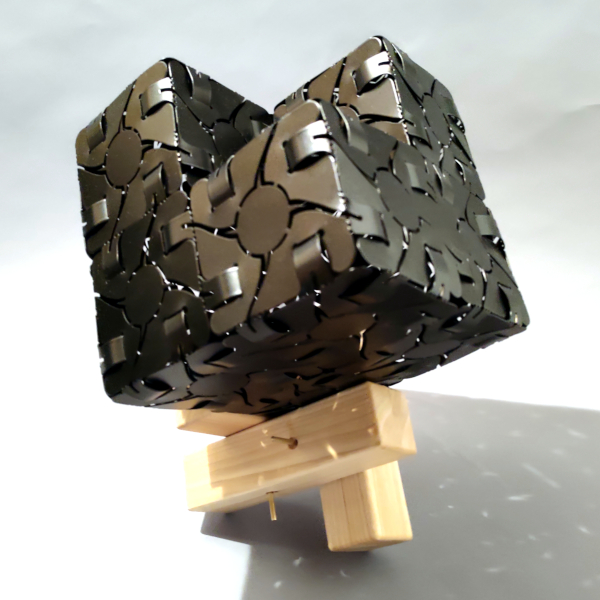
woven tile hexahedron
Copies of a single metal tile are woven together to form a cube with a concave vertex.
metatronic solid face (hexahedron)
A single shape shows four negative paths curving around the center where they would otherwise intersect.
isometric hex knot
Outlined knot segments are inlaid with copper wire. Each segment follows a path which eventually returns to its origin.
unicursal polygonal tercet
Stippled paint creates a unicursal knot design. The aggregation of dots suggests straight lines not present in the artwork. This single component is connected end to end with two rotated copies to create the knot. Doubling the individual segments of the knot design does not disrupt the over / under pattern that knot diagrams have.
metatronic solid (tetrahedron)
Three lines make a reversed knot pattern covering all faces of a tetrahedron, regardless of rotation (this same pattern can tile a plane).
projected isometric tercet knot
This is a double-lined, triple-component knot based on an isometric grid. The rules of knot making are slightly bent with the double line, but half the fun of making your own system is breaking its rules. It is “projected” is because its construction uses small wooden dowels to lift the individual pieces above the surface.…
tercet knot progression with self-similar encompassment
knots entangled with smaller versions of themselves
